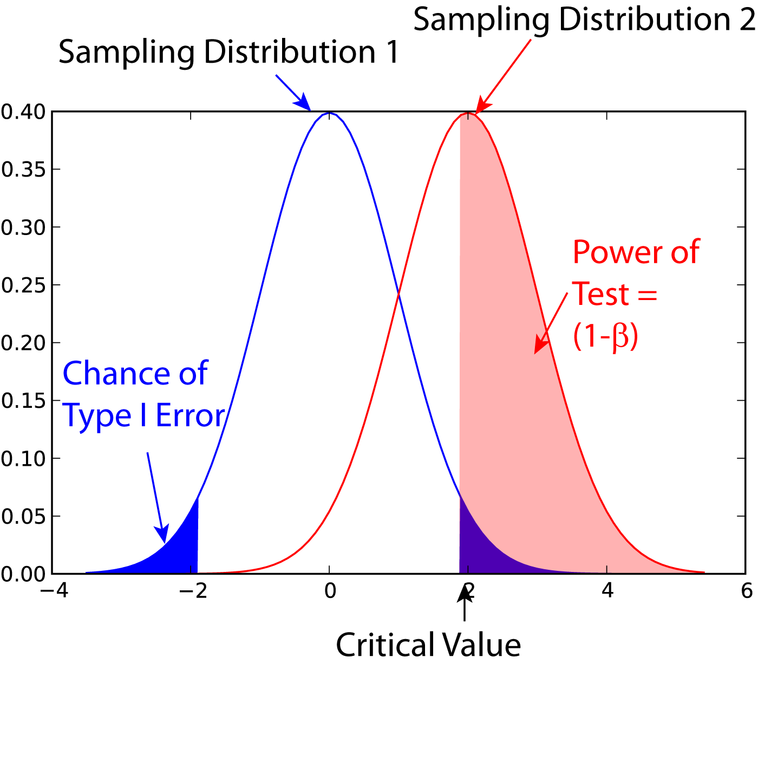
To estimate the number of patients needed for a clinical study, three factors are taken into account: the expected effect size of the intervention, the desired level of statistical significance, and the anticipated variability in the outcome measure.
The expected effect size
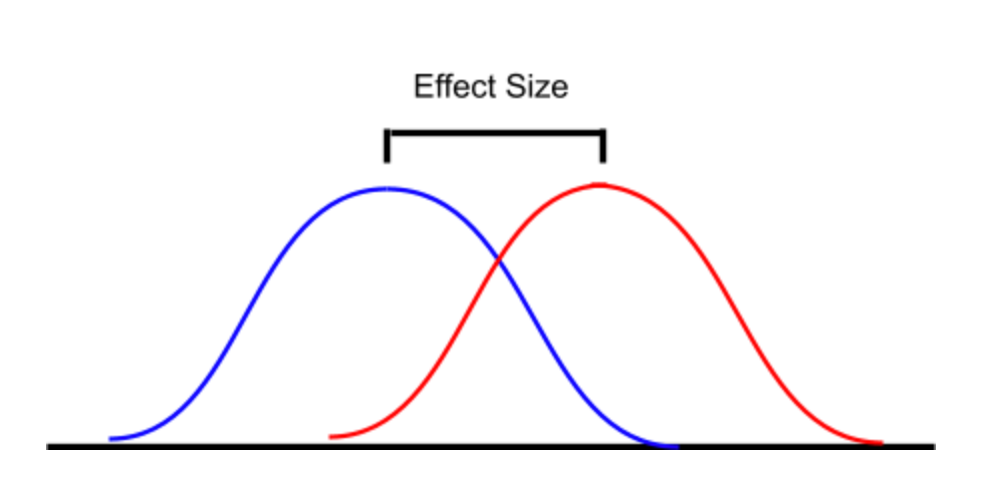
The expected effect size of an intervention is the anticipated magnitude of the effect that the intervention is expected to have on the outcome measure of interest.
Example : In a clinical study evaluating the effectiveness of a drug treatment, the expected effect size is the anticipated difference in the outcome measure between the participants receiving the drug and those receiving the placebo.
It matters to define the expected effect size, because it influences the sample size for a clinical study.
If one expects a large effect size, then it requires fewer samples to detect a statistically significant difference between the intervention and control groups.
The desired level of statistical significance
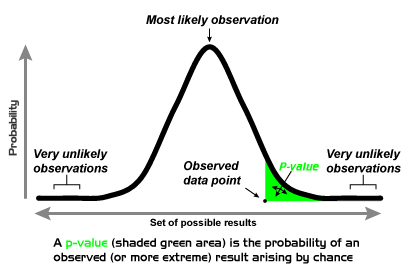
When doing the power analysis for a proposed clinical study, one typically sets the desired level of statistical significance to 0.05 (or 5%).
The percentage 5% refers to the chance that the results of the study are due to chance alone rather than being a true effect of the treatment being studied.
The choice of the level of significance is a balance between having a sufficiently large sample size to detect a true effect, and a not too large sample size to prevent wasting resources.
The anticipated variability in the outcome measure
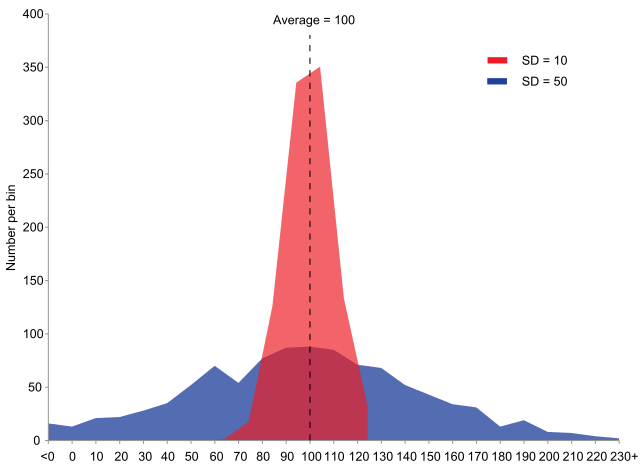
When doing the power calculations to calculate the size of the sample required to detect a true effect of the treatment being studied, the variability in the outcome measure is a required factor.
If the outcome measure is highly variable, then a larger sample size is needed to detect a true effect.
In the opposite, if the outcome measure is relatively consistent, then a smaller sample size may be sufficient.
Power analysis to determine the optimal sample size
It's a statistical technique that helps determine the sample size needed for a clinical study.
Power analysis involves estimating the variability in the outcome measure, the desired level of statistical significance, and the minimum detectable effect size.
Power analysis helps determine the optimal sample size for their study, ensuring that they have sufficient power to detect a true effect of the treatment being studied, while avoiding recruiting more participants than necessary.